Theoretical physics is a highly abstract discipline. Mathematics is its language, logic its method. But theoretical physicists are human, too, and our brains tire from the effort of thinking about things that hover on the edge of inconceivability. And so, to relieve the mental strain, we sometimes attach concrete images to the technical terms and mathematical symbols of our craft. Thus I think of electrons as fuzzy yellow tennis balls, of trajectories of photons as undulating blue lines, and of quarks as colored glass marbles. Our images, like those of painters, are derived from the simple things we see in the world around us. That isn't really surprising, for physics, like all creative endeavors, engages the imagination, and the word "imagination" comes, not by coincidence, from "image."

Physics conjures up images and, conversely, images stimulate thoughts about physics. The paintings of Ronald Davis are especially inviting to this kind of meditation because they display just the right blend of realism and abstraction. In particular, it seems to me that Davis's work can be seen as a metaphor for the way theoretical physicists think about the world. To learn about the workings of a physicist's mind, without having to delve into the actual theories, we can do no better than to turn to Albert Einstein, who recorded some of his profound insights into his own mental processes. Davis's work, through its imagery, helps us to get a glimpse of the great physicist's thinking. At the same time, Einstein's way of seeing the world illuminates Davis's art.

Einstein was primarily a visual thinker. He rarely thought in words at all, and mathematics did not come naturally to him-he used it only to the extent that he had to. The special theory of relativity, for example, is couched in terms of high school algebra, while the later, much more sophisticated theory of gravity requires a formalism that took Einstein ten arduous years to learn. The basic objects of his thinking were visual images. Gerald Holton, in an essay entitled "On Trying to Understand Scientific Genius," quotes Einstein's own description of his mental activity in words that apply equally well to Davis's work:

What, precisely, is "thinking"? When, upon reception of sense-impressions, memorypictures emerge, that is not yet "thinking." And when such pictures form a series, each member of which calls forth another, this too is not yet "thinking." But when a certain image turns up in many such series, then-precisely by its return-it becomes an ordering element--a concept.... It is by no means necessary that a concept must be connected with a recognizable sign or word.... All our thinking is of this nature of a free play with concepts.

And elsewhere Einstein elaborates:

This combinatory play seems to be the essential feature of productive thought before there is any connection with logical construction in words or other kinds of signs (such as mathematical symbols) which can be communicated to others. The elements mentioned above are, in my case, of visual and some of muscular type. Conventional words or other signs have to be sought for laboriously only in a secondary stage, when the associative play is sufficiently established.

The term "play" occurs often in Einstein's writings about the creative process. He played with concepts the way a dog worries a bone and the way Davis plays with the visual possibilities inherent in an arch or a box. But for both Einstein and Davis play is serious. The intensity with which Einstein juggled the same sparse set of concepts--elativity, symmetry, continuity, atomicity--for an entire lifetime is echoed in the singleminded concentration with which Davis explores his own set of themes. This may be play, but the approach is not playful. Davis and Einstein look at the world with childlike, eyes, but they are the eyes of grave and deeply thoughtful children.

This play with concepts, for both men, is guided by a simple purpose: to get it right. Einstein, when he formulated special relativity, did not set out to revolutionize physics. All he had wanted to do was reconcile a seemingly trivial inconsistency in classical physics. As a teenager, he tried to imagine what he would see if he rode along a beam of light at its own speed, and later he found out that mechanics and optics gave different answers to the question. It wasn't a very pressing problem but, as in everything else he did, Einstein was deter mined to get it right. Davis, too, is concerned with simple objects, and the seemingly inconsequential problems they pose. Where do the lines meet? Is the shadow here a little darker, or should it be lighter? How do the planes overlap? Are they parallel, or not? These are questions that face all painters, but because he concentrates on them more sharply, Davis has to answer them more precisely And invariably he gets them right. Right, not in the simplistic sense of verisimilitude, but by the more exacting standards of artistic integrity. The cogency of Davis's work reminds us of the ease with which Einstein's theory of 1905, with the famous E = mc², in spite of its strangeness, convinced the majority of his colleagues that it must be right.

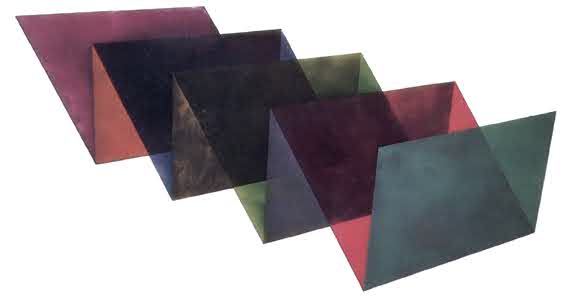
Sawtooth. 1970, 64 1/2 x 138 inches, polyester resin and fiberglas.

Davis' painted objects may be seen as metaphors for Einstein's images or concepts. For the fiberglass pieces this relationship is straightforward. As a child learning geometry, Einstein felt that "the objects with which geometry deals seemed to be of no different type than the objects of sensory perception, which can be seen and touched." Euclid's constructions were, for Einstein, tangible objects. Yet they are not simple objects that can be easily described in words. Like Davis's objects, they are fine and subtle things in which solidity and palpability compete with an essential ineffability. One can imagine Einstein, as a boy, seeing the entire proof of the Pythagorean theorem appear before his inward eye in the form of Davis's Sawtooth (1970). It is the proof of the theorem, not merely the statement, that so appears. The statement is a simple fact, easily verbalized and memorized. The proof, on the other hand, is an intricate process that requires active thinking: "First you draw this auxiliary triangle, and then that one, and then you notice their connection In looking at Sawtooth, your eye and brain are similarly compelled into action, comparing, measuring, visualizing hidden spatial relationships, jumping from three dimensions to two and back again and, finally, with a sigh of satisfaction, concluding that it is right. Just like the Pythagorean theorem.