I was looking at the packing orders of various shapes and how they fit into other shapes and wondering of course why there's no Mac software for circle packing since these "gaskets" as they call them — are suddenly starting to be a hot item in mathworld.

The shapes I used in the hilbert-reiteration were 2D "gaskets" of the hilbert curve space-filling set. Like — OK, you've got these shapes and orders and particles and all like that but if you _subtract_" them from everything thing that is, there's more _is_ left than there was stuff you defined so I've always been interested in what was left over after you thought you had thought you had thought of everything like, why am I sawing through my finger with this powerful? And why does my ladyfriend continue to live with me? (I am not worthy...) Things like that.

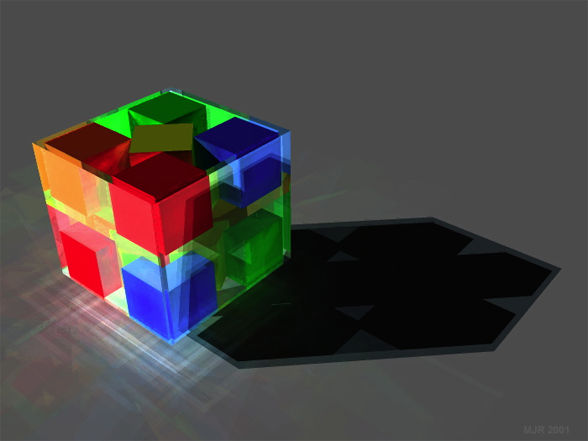
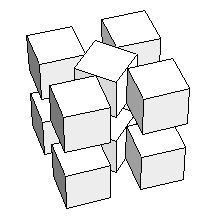
So anyway there's these other nerds somehow get PAID to think about this stuff and one of the things one of them put up on a site caught my eye was the packing order of cubes in cubic space (which I guess I've been thinking about for some time now) and there are only so many ways you can fill a cubic space of a given size with cubes of a given size.

Which I thought was cool so I made a model (more of less) of it and jazzed it up a bit with an open top & bottom plexibox to show the volume of its cubeness and then rendered it more ways for more time than I should have considering I'm going broke — and came up with this.

Which I finally liked well enough to send along to you.